Course on Complex Analysis Vanderbilt 2017
Course on Complex Analysis Vanderbilt 2017
Syllabus:
Complex integration, calculus of residues, harmonic functions, entire and meromorphic functions, conformal mapping, normal families, analytic continuation,elliptic functions, iteration of rational maps.
Course Description.
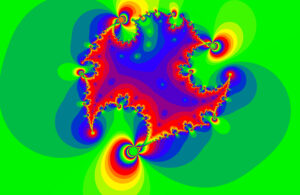
The course is a first graduate course in complex analysis. We will be begin by covering the usual topics of an undergraduate course, going quite quickly but with occasional in depth visits to significant results. Thus we will carefully prove Cauchy’s formula and give a treatment of the residue formula over general contours. Topics covered in this way will include the maximimum modulus theorem,harmonic functions and harmonic conjugates, Schwarz reflection principle, the argument principle, Taylor and Laurent series, Rouché’s theorem,branches, a little analytic continuation, Schwarz lemma. Then we will introduce some new topics including normal families building up to the first major goal of the course, the Riemann mapping theorem that states that any simply connected domain in the complex plane ℂ, different from ℂ is conformally equivalent to the unit disc. According to the time available we will look at elliptic functions including at least the Weierstrass ℘ function. Finally as a special topic we will investigate iteration of rational functions where we meet the Julia, Fatou and Mandelbrot sets and learn how to understand pictures like:
Homework
Throughout the course emphasis will be placed on examples and homework will be assigned regularly. Look for homework assignments HERE
Second homework assignment due Friday September 8
Third homework assignment due Friday September 15
Fourth homework assignment due Friday September 22
Fifth homework assignment due Friday September 29
Sixth homework assignment due Monday October 16
Seventh homework assignment due Friday October 20
Eighth homework assignment due Monday November 6
Tenth homework assignment due Monday November 6
As discussed in class there will be projects – max of 3 page writeup plus an in-class presentation. Here is the list of potential topics <\h3>
Potential projects I wanted to give you all some feedback on the presentations in the last week. It was a very difficult task and I somewhat deliberately didn’t give you much guidance, I wanted to see what you would make of it. I think you all realised that preparing and giving a talk of less than 20 minutes is challenging. You have to think very carefully about what you want to say and say only what is necessary to achieve that goal. Adding things that “seem important” just uses up valuable time. Apart from that I was looking for something that I didn’t see too much of and that was “getting to the essence of the proof/idea”. I wasn’t expecting you to understand proofs in detail but I was hoping to see that you had understood at some level what was making it work. For instance in the case of the modular forms there was the result that the numbers of zeros and poles in a fundamental domain are equal. I would have liked to hear something like “by integrating around the boundary as for elliptic functions, but one has to worry about infinity” or words to that effect. Or for the Riemann zeta function, what was never given was any idea of how one analytically extends. This involves an integral transform and the functional equation which never reared its head. For the uniformisation theorem there was no hint of how the combinatorics of a triangulation might yield the 3 different possibilities for a simply connected surface. The quasiconformal topic was harder than the others and perhaps because of that the team working on it had sought some kind of intuitive understanding in the face of overwhelming technical difficulties. In general I thought I would offer some advice on how to quickly get something out of reading a theorem which one has to know on some level. The first job is simply to spend some time thinking about how one might actually prove it oneself. This is very useful and the amount of time spent doing that should depend on how much one needs to know. If it’s a serious theorem you won’t actually be able to prove it just like that but the next job is to start looking at the proof and comparing it to the ideas you had. It is pretty rare that there will be empty intersection and what intersection there is will help you massively in understanding the proof. You might even get the “aha I almost had it” feeling and go away thinking that you have really got the proof. Anyway, I enjoyed listening to your presentations and I had a good time teaching the course over the course of the semester. I wish you all the best of success in your mathematical careers! Vaughan
Arzela Ascoli on equicontinuous families
Branches and Riemann surfaces for inverse functions and relations.
Weierstrass function inverts elliptic integral
Meromorphic functions with prescribed principal parts
Iterating rational functions on the Riemann sphere
-
Textbook:
the text for the course will be “Complex Analysis” by Stein and Shakarchi. I will also use Sarason’s “Notes on complex function theory.”
Midterm, grades
So that I have some idea how much students are understanding we will have a midterm in class on Thursday 28 September.Otherwise grades will be assigned on the basis of a short (2-3 pages) essay on a topic not covered explicity in the course, e.g. “The Riemann Zeta function.” Topics may be proposed by students or selected from a list that I will give. The student is supposed to investigate the literature on the topic and the essay will be the result of that investigation. I encourage students to work in groups of at most 3, and will be happy to discuss progress on the essay with a group.
Office hours
Tentative office hours are Tuesday and Thursday from 3pm to 4pm and may also be arranged by appointment. My office is SC1413
