Multiscale Modeling of Wave Propagation in Viscoelastic Phononic Crystals and Acoustic Metamaterials

Research Sponsor:

US National Science Foundation

Investigators:

Ruize Hu and Caglar Oskay

Motivation

• Phononic crystals and acoustic metamaterials exhibit extraordinary capability in controlling

  acoustic and elastic waves by manipulating band gaps that forbid waves to propagate within

  targeted frequency ranges.

• Modeling of transient wave propagation in these composites using direct numerical simulations is computationally prohibitive for structural design and analysis.

Objective

• Develop a multiscale computational framework for efficient modeling of transient wave propagation in periodic viscoelastic composites, capturing wave dispersion and bandgap phenomenon.

• Investigate the effects of microstructural morphology, elastic and viscoelastic constituent material properties on the overall wave attenuation behavior.

   

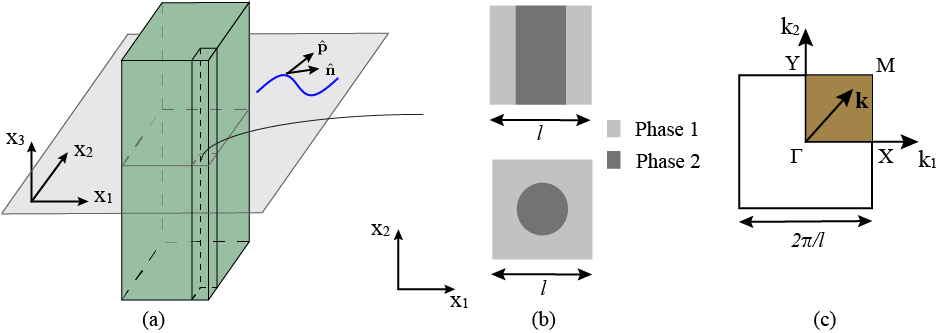
Spatial-temporal nonlocal homogenization model (STNHM)

High order asymptotic expansion

• Critical in extending the classical homogenization theory to short wavelength regime.
• Introduce spatial nonlocal terms in momentum balance equations at successive orders.

Construction of gradient-type nonlocal governing equation

• Derive temporal nonlocal terms in high symmetry directions of the first Brillouin zone.
• Nonlocal model parameters are uniquely determined by minimization of asymptotic residual.

Nonlocal effective medium model (NEM)

• Governing equation in the second order form with nonlocal features retained by the frequency-dependent nonlocal effective moduli tensor.
• Simulation of transient wave propagation without need of high order boundary conditions.

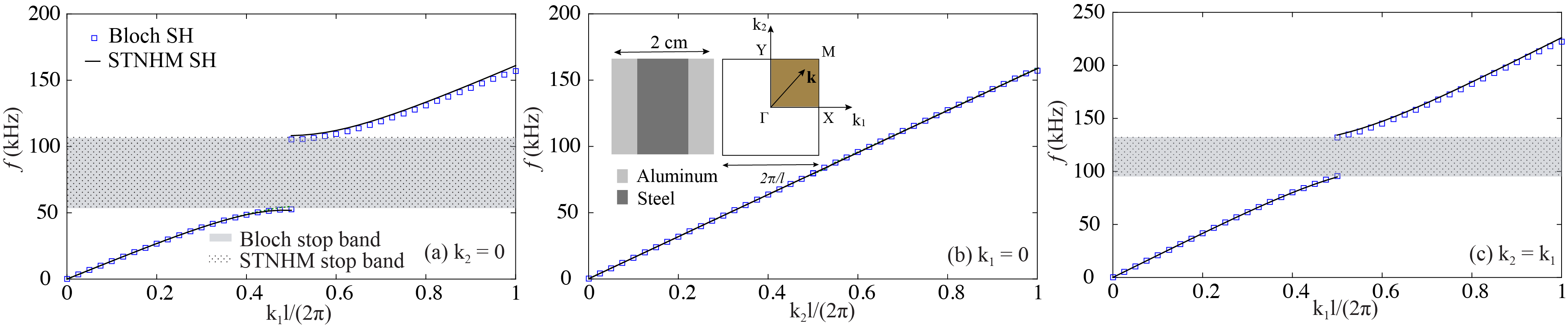
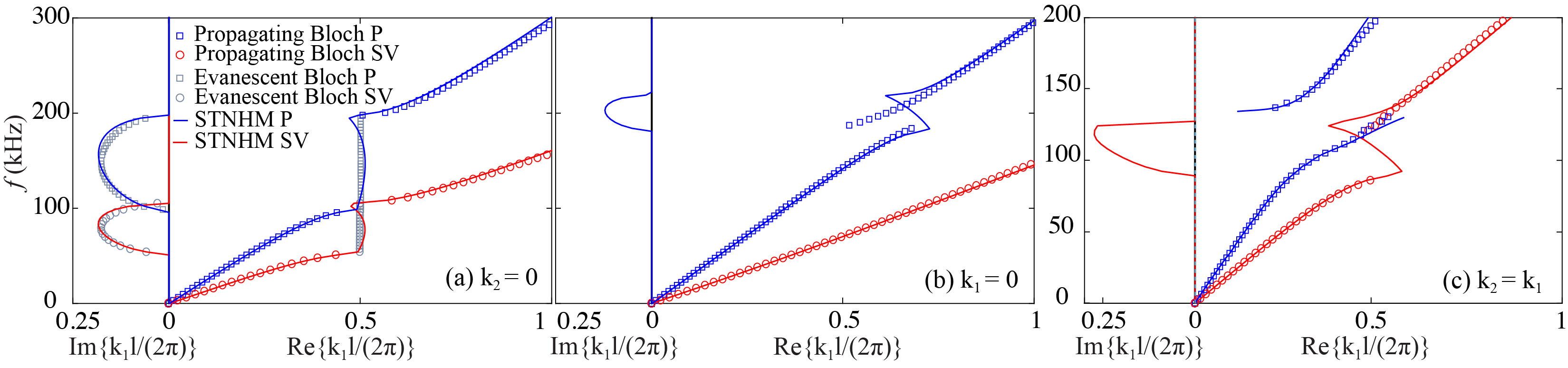
Dispersion relation

• Captures the acoustic branch, the stop band and the first optical branch in high symmetry directions.
• Evanescent wavenumber is predicted within the stop band.

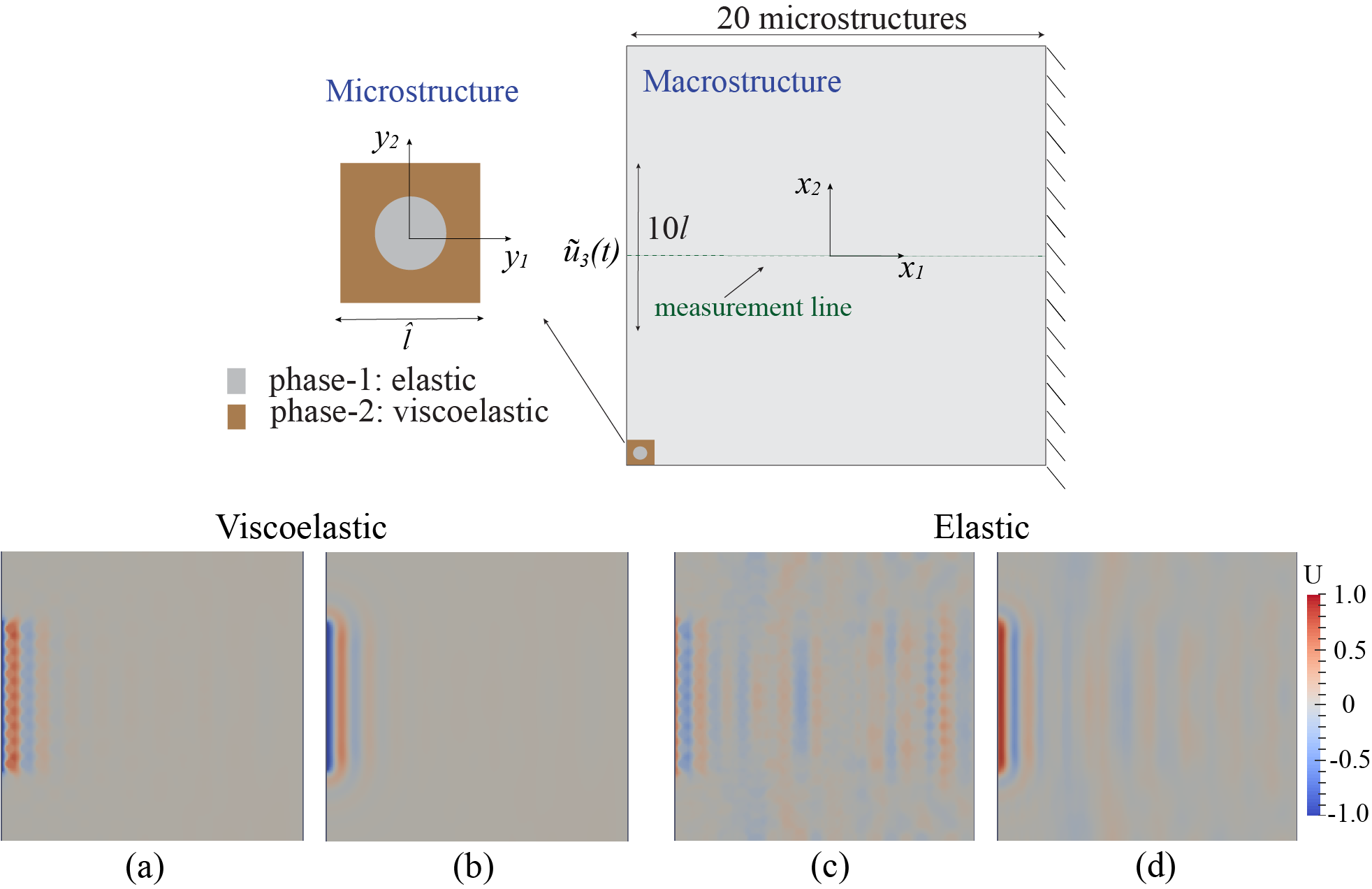
Anti-plane shear wave 

In-plane wave

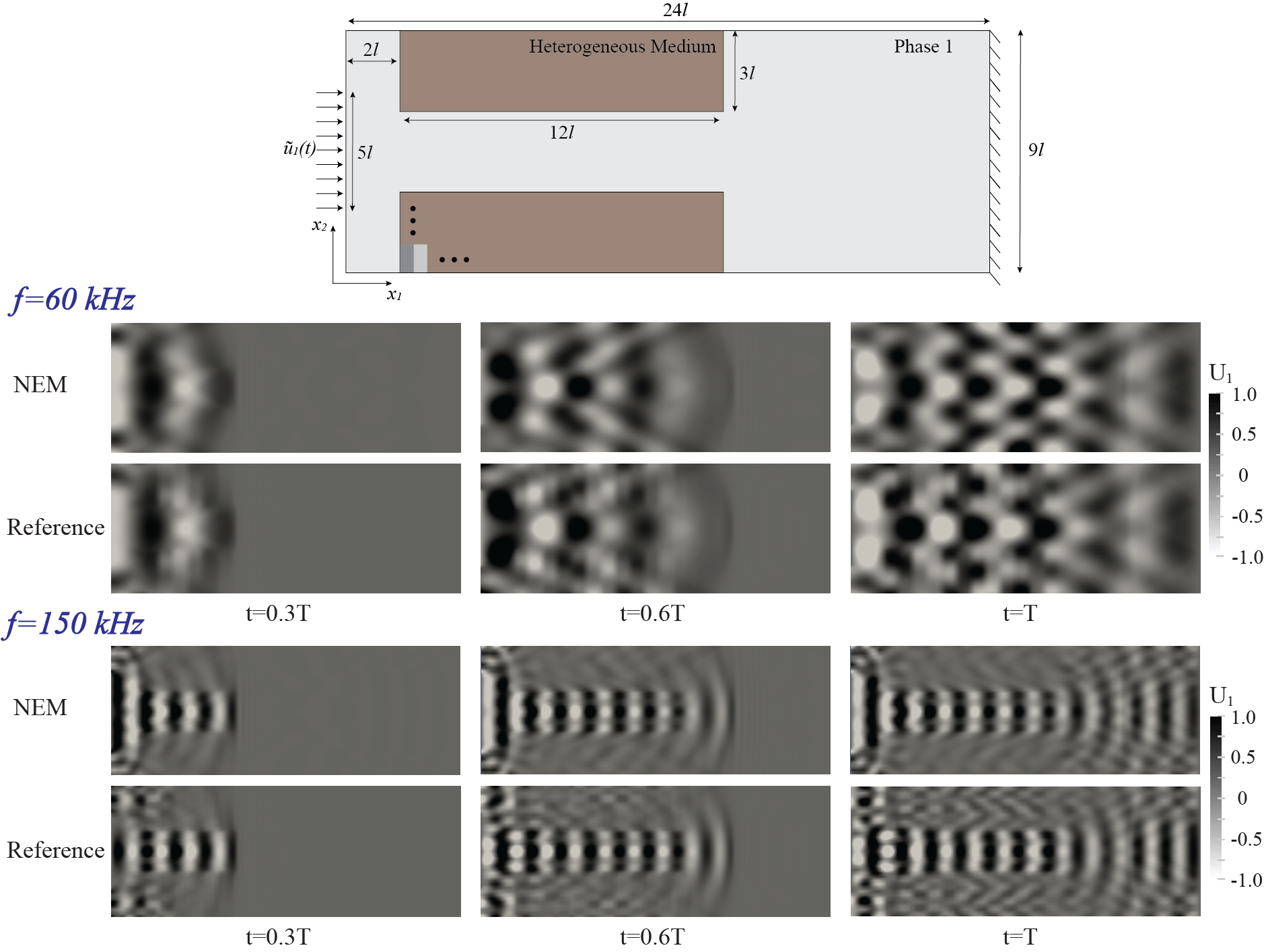
Transient wave propagation

In-plane elastic waveguide modeling

• Wave dispersion occurs at relatively low frequency.
• Highly confined wave propagation when wave frequency is within the stop band.

 

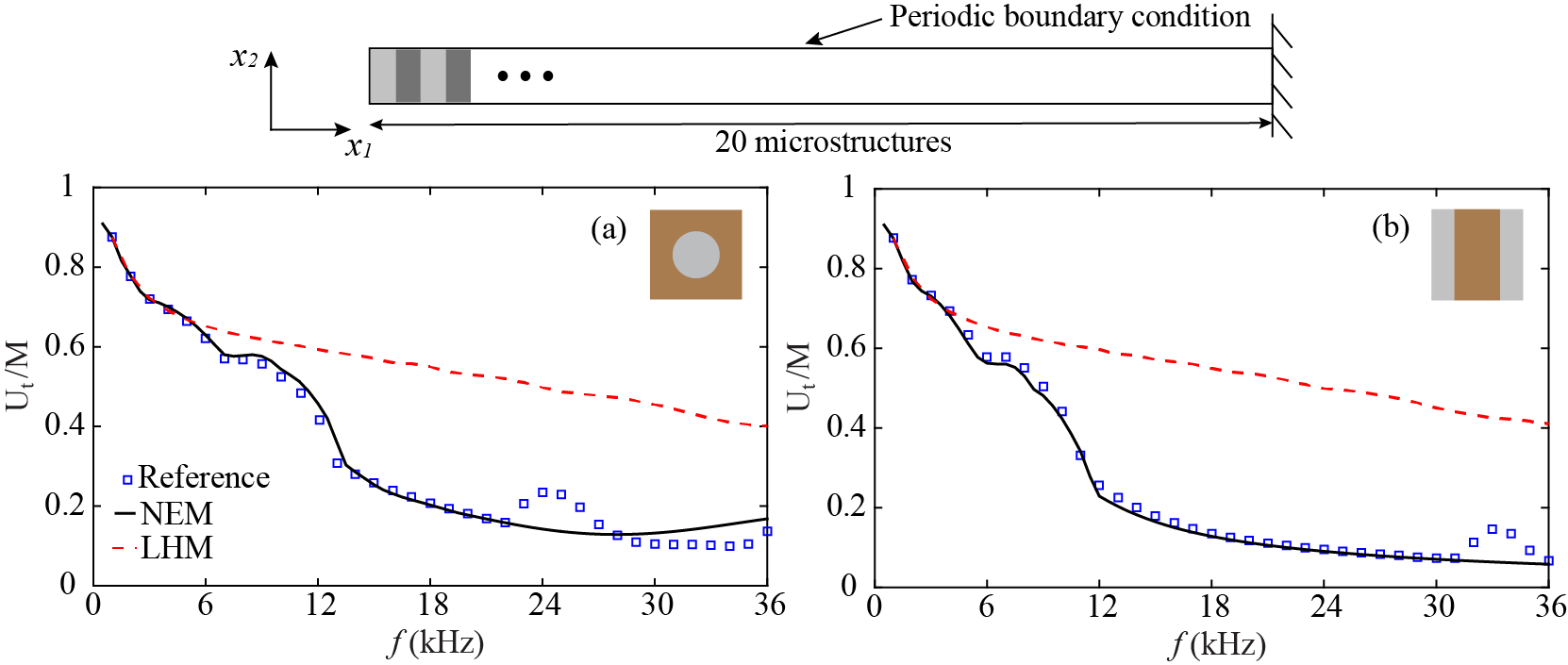
Anti-plane shear wave propagation in viscoelastic phononic crystal

• Nearly complete attenuation due to band gap formation and viscoelastic dissipation.
• Stronger attenuation compared to elastic phononic crystal.

• Compared to having viscoelastic dissipation only (LHM), band gap formation significantly improves wave attenuation.
• Layered microstructure has wider stop band and stronger wave attenuation within the stop band.