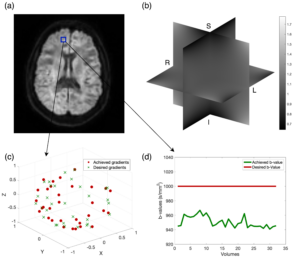
In diffusion MRI, gradient nonlinearities cause spatial variations in the magnitude and direction of diffusion gradients. Studies have shown artifacts from these distortions can results in biased diffusion tensor information and tractography. Here, we investigate the impact of gradient nonlinearity correction in the presence of noise. We introduced empirically derived gradient nonlinear fields at different signal-to-noise ratio (SNR) levels in two experiments: tensor field simulation and simulation of the brain. For each experiment, this work compares two techniques empirically: voxel-wise gradient table correction and approximate correction by scaling the signal directly. The impact was assessed through diffusion metrics including mean diffusivity (MD), fractional anisotropy (FA), axial diffusivity (AD), radial diffusivity (RD), and principal eigen vector (V1). The study shows (1) the correction of gradient nonlinearities will not lead to substantively incorrect estimation of diffusion metrics in a linear system, (2) gradient nonlinearity correction does not interact adversely with noise, (3) nonlinearity correction suppresses the impact of nonlinearities in typical SNR data, (4) for SNR below 30, the performance of both the gradient nonlinearity correction techniques were similar, and (5) larger impacts are seen in regions where the gradient nonlinearities are distinct. Thus, this study suggests that there were greater beneficial effects than adverse effects due to the correction of nonlinearities. Additionally, correction of nonlinearities is recommended when region of interests are in areas with pronounced nonlinearities