Tensor Interpolation by Concise Local Estimation of Anisotropy and Rotation
A. Landman, P-L. Bazin, and J. L. Prince. “Tensor Interpolation by Concise Local Estimation of Anisotropy and Rotation”, In Proceedings of the Workshop on Computational Diffusion MRI at the 11th International Conference on Medical Image Computing and Computer Assisted Intervention, New York, NY, September 2008.
Full Text:http://citeseerx.ist.psu.edu/viewdoc/download?doi=10.1.1.701.4262&rep=rep1&type=pdf#page=265
Abstract
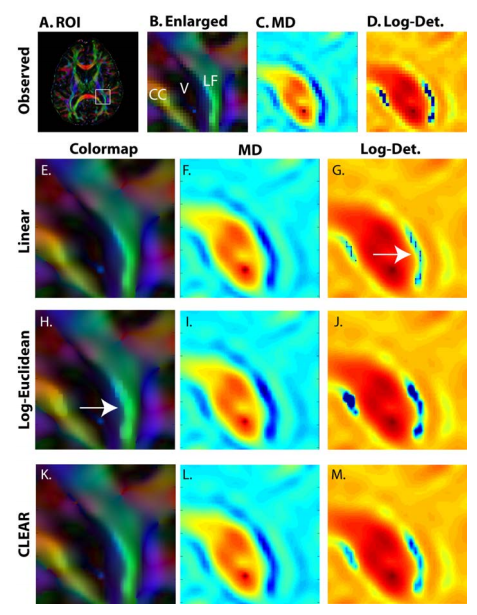
In diffusion tensor imaging, tensors represent the oriented probability of intra-voxel water diffusion. These positive definite, symmetric matrices form a non-Euclidean space which poses challenges for interpolation and analysis. In particular, most tensor interpolation schemes have a non-linear effect on the clinically important tensor attributes of fractional anisotropy and mean diffusivity, resulting in tensor “swelling.” We propose a new interpolation method that explicitly preserves userspecified interpolation of fractional anisotropy and mean diffusivity, while independently interpolating diffusion directions. The approach leads to an efficient technique that is practical for routine use.